How does the collective behavior of biological tissues emerge from individual cellular behavior? Tissues and cellular aggregates display a rich variety of complex, non-equilibrium phenomena, and understanding the impact of microscopic cellular interactions on mechanical tissue properties will help to better understand processes ranging from multicellular development to wound healing to cancer mechanisms. For example, recent experiments have revealed collective rigidity transitions in dense tissues intimately related to an observed change in cell-scale geometric and material parameters, and such transitions have been interpreted through the lens of glass-like and jamming transitions. Strikingly, although in particulate systems the athermal jamming transition is distinct from both thermal and non-equilibrium dynamical glass transitions, it has been suggested that these may coincide in models for dense biological tissues.

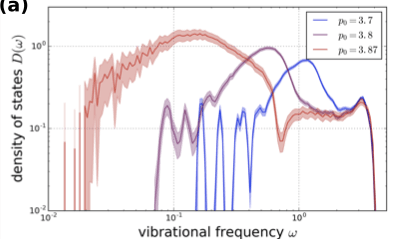mode plot “Vertex” and “Voronoi” models are two variations on a similar idea — modeling confluent tissue by a polygonal/polyhedral decomposition of space, and writing down an energy functional that depends on the resulting geometry of the cells. Each have used, at times as if they were interchangeable, to try to understand fluid-to-solid transitions in disordered cell configurations. Recently we have tried to put this scenario on much firmer theoretical footing, in particular with respect to the existence of an underlying jamming-like transition for vertex and Voronoi models governed by a standard quadratic energy functional (where each cell has a harmonic spring tied to a preferred area and a preferred perimeter).

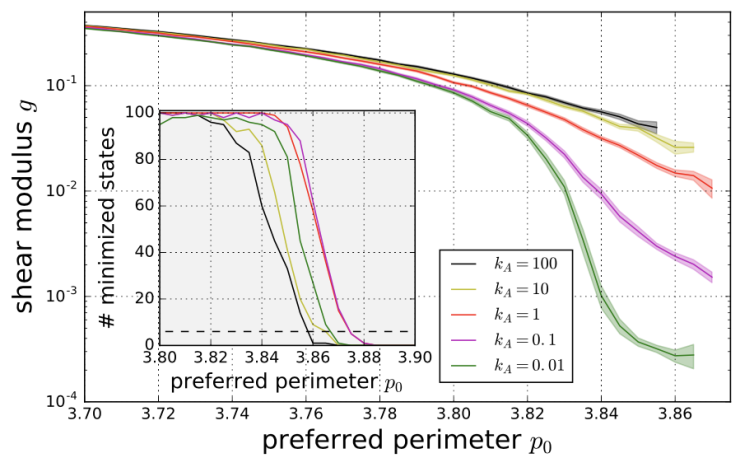shear modulus plot In particulate models the jamming transition can be helpfully interpreted through the lens of “Maxwell” or constraint counting , in which one considers the balance between a model’s degrees of freedom (d.o.f.)and constraints. For instance, in soft sphere systems a small change in the density can lead to large changes in the number of contacts, and the jamming transition occurs when the system is marginal, with contacts (constraints) and degrees of freedom exactly balanced. In this framework one would expect clear differences between classical jamming models and the various vertex and Voronoi models. In the 2D vertex and 3D Voronoi models the number of d.o.f. is always larger than the number of constraints represented just by area and perimeter springs; these models are under-constrained, and the system is rigidified by the collective onset of residual stresses. In stark contrast, the 2D Voronoi model always marginal, and a constraint-counting perspective would predict that it lacks any athermal rigidity transition. In light of this, hypothesized connections between the 2D vertex and Voronoi models, and their jamming and glass transitions, are especially surprising.

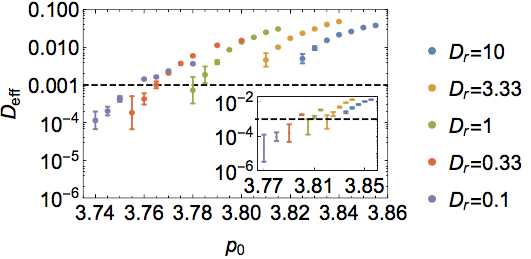Effective diffusion We confirm this scenario with in-depth numerical studies of both the disordered ground state configurations of the models and also by looking at the very-low temperature dynamics. We show that the Voronoi model, surprisingly, has no unjamming transition, and existing data can be rationalized in terms of a nearby avoided transition (in the limit that either the Voronoi constraints are relaxed or the area springs are taken to have zero strength). As an object of future study, we thus find a model whose zero-temperature limit is always marginal, suggesting that it may possess very interesting finite-temperature glassy behavior.